What is the Rule of 72 Calculator (Time to Double the Money Calculator)

The rule of 72 calculator or time to double the money calculator is a simple online tool that uses interest rates and calculates the number of years required to double your money. Alternatively, you can also find the interest rate required to double your money when the number of years is given

Steps:

1. Click the number of years and the annual interest rate which you expect for your money and enter, the calculator will show the number of years required to double your amount.
2. Click Interest rate and enter the number of years, the calculator will show the rate of interest required to double your amount

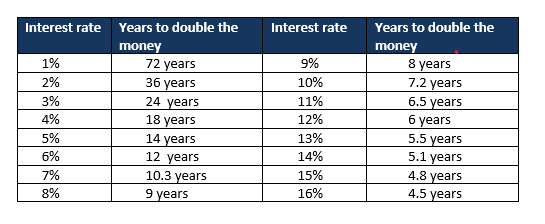
Sample illustration of The Rule of 72 (Time to double the money table)

 

What is the ‘Rule of 72’ Formula?

The rule of 72 Formula is given by

         T = 72 ÷ R

          where,

          t = time required to double the money,

          R = Expected rate of interest,

          Alternatively, we can also calculate the Rate of interest required to double your money by the below formula

           R = 72 ÷ T                        

How to find the time to double the money?

The compound interest formula is used to derive the formula of ‘Rule of 72’. As we know the formula for compound interest is

A = P (1 + r)^t

Where A is the final amount, P is the principal amount, r is the rate of interest rate per period in decimal form and t is the number of periods.

Now when we rewrite the formula as

2P = P (1 + r)^t

And divide by P into both sides we get,

(1 + r)^t  = 2

Now take the natural log on both sides, we get

t × ln (1+r) = ln (2),

In the above equation, we get the equation for t as

t = ln (2) / ln (1+r)

or

t = ln (2) / r × r / ln (1+r)

Now solving the equation by taking natural log values we get the value of t as,

t = 72/r